| Authors: | Griton, L.; Pantellini, F.; Meliani, Z. |
Abstract: We present magnetohydrodynamic simulations of a fast-rotating planetary magnetosphere reminiscent of the planet Uranus at solstice, that is, with the spin axis pointing to the Sun. We impose a 10 times faster rotation than for Uranus, in order to emphasize the effects of rotation on the magnetospheric tail without the need of an excessively large simulation domain while keeping the qualitative aspects of a supersonic magnetized solar wind interacting with a fast-rotating magnetosphere. We find that a complex helical Alfvénic structure propagates downstream at a velocity exceeding the plasma velocity in the magnetosheath. Similarly, the reconnection regions, which mediate the interaction of the planetary magnetic field and the interplanetary magnetic field, do also form a helical structure with the same downstream velocity but a 2 times larger pitch. We speculate that the magnetic field of the helical structure connected to the interplanetary magnetic field asymptotically reduces the phase velocity of the helical structure toward the tailward velocity in the magnetosheath. For our simulations we use the MPI-AMRVAC code which we enhanced with a time-dependent background magnetic field in the splitting of the magnetic field.
©2018. American Geophysical Union. All Rights Reserved.

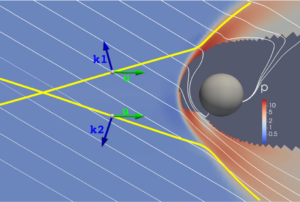
 See the paper for the legend of this figure. (c) 2015 Elsevier Ltd
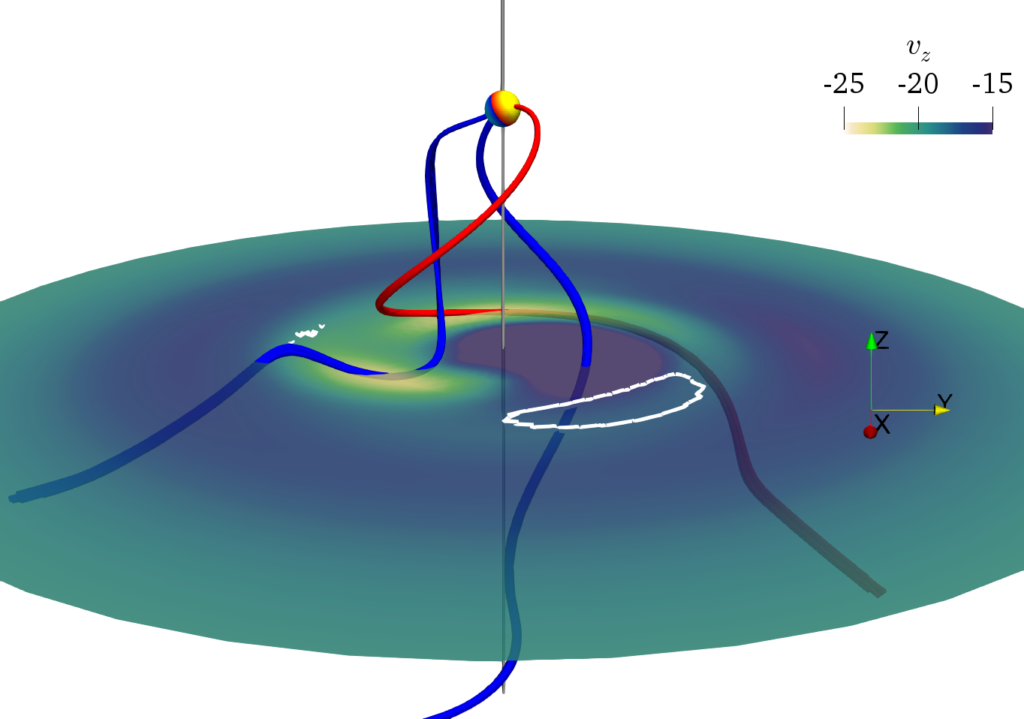
See the paper for the legend of this figure. (c) 2015 Elsevier Ltd